Today I have an update on using the Colour Factor set. For the rundown on how this maths teaching tool helps kids visualise numbers as something concrete and dynamic, have a look at my earlier post on these coloured rods. My daughter’s teacher at the local Spanish school has used them for learning addition and subtraction. But their wonder, for me, lies in how far you can take maths using them with even fairly young children. The author Máire Mullarney talks about overhearing her two boys aged around five and seven discussing whether or not 17 was a prime number, and when you actually play with the blocks, you can see how that would be possible, without your kids having to be child prodigies!

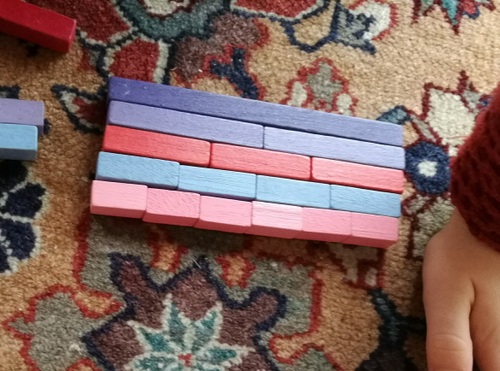
We haven’t had the blocks out much in the past six months so I was happy to take advantage of my littlest having a nap on a quiet rainy day to tip the box of blocks on the rug and get playing with his five-year old sister. We played at making patterns with them, and I laid out the following:

This prompted her to ask why some were similar colours. I decided to do the odd “stairs” and even “stairs” with her. I checked first that she already had a clear idea of odd and even numbers. She understood them as sequences of alternate numbers and also that evens were multiples of two. In other words, in colour factor terms, you can’t make a row of pink twos the same length as an odd number. To show the relationship of even numbers to each other, which is the role of the warm reddish colours, we grouped the even number blocks as stairs, noting that the difference in length of adjacent rods is equivalent to two, not one as in the full set of stairs, and noting the colours.

Then I asked her to match the length of various even rods using a row of rods of a different colour. Seaton Pollock called these single-colour rows “trains”. This shows tangibly the role of factors because of their physical size, and the colours underline the message. Next we set about laying out the factors of twelve. We began with a mauve rod representing twelve, alongside two sixes. I asked her to add another single-colour row which would be the same length and she chose six pink rods, or twos. Next she added four threes. Then I introduced the word “factor”. It clicked that the factors are represented by colours, hence the name Colour Factor Set! I can honestly say I would never have dreamt of discussing factors with a five year old just using pen and paper. But with the rods it comes easily.

Most of colour factor is about learning on the basis of what is physically visible in the blocks, which is why it works so well with little kids. However, since she has already done quite a lot of simple sums on paper at school I was ready to bring in a written format. I wrote out the following to show both ways of presenting the facts: 6 + 6 = 12 2 x 6 = 12 She digested that.

 

Then I wrote out the “trains” we had before us in the order we had made them: 1 x 12 = 12 2 x 6 = 12 6 x 2 = 12 4 x 3 = 12. Then I asked if she wanted to know a trick to find yet another factor, and we discussed how 2 x 6 (two violets) came to the same as 6 x 2 (six pinks). This made immediate sense to her. She quickly realised that above the row of four light blue rods (4 x 3) she could make a train showing 3 x 4 with the scarlet blocks, and finally a row of twelve whites (12 x 1 = 12). Then she decided to write these on paper herself, adding to our list with a triumphant flourish.

Finally, I returned to my original rows and told her one set was missing from the pattern. She immediately completed it like this:

You can get a secondhand copy of Máire Mullarney’s book Anything School Can Do, You Can Do Better for a song off Amazon, and Colour Factor Sets come up on eBay from time to time. Don’t worry if a few of the smaller blocks are missing from these vintage pieces, the set will still be perfectly usable.

---

las regletas – rods

números pares – even numbers

números impares – odd numbers

menos – minus

más – plus

dos veces cuatro igual a ocho – 2 x 4 = 8